 T
T
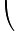

 a b c
a b c 

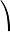
 =
= a−b 3a+b+c 2a+2b+c −2a−6b−2c
a−b 3a+b+c 2a+2b+c −2a−6b−2c 
From A First Course in Linear Algebra
Version 2.01
© 2004.
Licensed under the GNU Free Documentation License.
http://linear.ups.edu/
Summary Domain is column vectors, codomain is matrices. Domain is dimension 3 and codomain is dimension 4. Not injective, not surjective.
A linear transformation: (Definition LT)
 T T 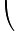   a b c a b c   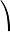  = = a−b 3a+b+c 2a+2b+c −2a−6b−2c a−b 3a+b+c 2a+2b+c −2a−6b−2c  |
A basis for the null space of the linear transformation: (Definition KLT)
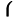  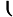   −1 −1 4 −1 −1 4   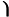 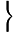 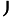 |
Injective: No. (Definition ILT)
Since the kernel is nontrivial Theorem KILT tells us that the linear transformation is
not injective. Also, since the rank can not exceed 3, we are guaranteed to have a
nullity of at least 1, just from checking dimensions of the domain and the
codomain. In particular, verify that

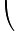

 2 1 3
2 1 3 

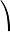
 =
= 1 10 9 −16
1 10 9 −16  T
T
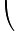

 0 −1 11
0 −1 11 

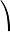
 =
= 1 10 9 −16
1 10 9 −16 
This demonstration that

 0 −1 11
0 −1 11 
 z =
z =
 2 1 3
2 1 3 
 +
+
 −2 −2 8
−2 −2 8 
 =
=
 −2 −2 8
−2 −2 8 
 ∈K
∈K T
T and
and so the vector
A basis for the range of the linear transformation: (Definition RLT)
Evaluate the linear transformation on a standard basis to get a spanning set for
the range (Theorem SSRLT):
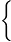  1 3 2 −2 1 3 2 −2    −1 1 2 −6 −1 1 2 −6    0 1 1 −2 0 1 1 −2  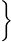 |
If the linear transformation is injective, then the set above is guaranteed to be linearly independent (Theorem ILTLI). This spanning set may be converted to a “nice” basis, by making the vectors the rows of a matrix (perhaps after using a vector reperesentation), row-reducing, and retaining the nonzero rows (Theorem BRS), and perhaps un-coordinatizing. A basis for the range is:
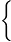  1 1 0 2 1 1 0 2    0 1 1 −2 0 1 1 −2  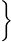 |
Surjective: No. (Definition SLT)
The dimension of the range is 2, and the codomain
(
To be more precise, verify that  2 1 −1 3
2 1 −1 3  ∉ℛ
∉ℛ T
T

 2 1 −1 3
2 1 −1 3 

Subspace dimensions associated with the linear transformation. Examine parallels with earlier results for matrices. Verify Theorem RPNDD.
Invertible: No.
Not injective (Theorem ILTIS), and the relative dimensions of the domain and
codomain prohibit any possibility of being surjective.
Matrix representation (Definition MR):
 C =
C =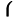

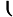

 1 0 0
1 0 0 



 0 1 0
0 1 0 



 0 0 1
0 0 1 

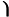
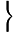
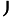 =
=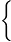
 1 0 0 0
1 0 0 0 

 0 0 1 0
0 0 1 0 

 0 1 0 0
0 1 0 0 

 0 0 0 1
0 0 0 1 
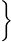 =
=



 1 2 3 −2 −1 2 1 −6 0 1 1 −2
1 2 3 −2 −1 2 1 −6 0 1 1 −2 



