Span of a Set of Column Vectors
Given a set of vectors
 u1
u1 u2
u2 u3
u3 …
… up
up
 S
S
 u2
u2 u3
u3 …
… up
up From A First Course in Linear Algebra
Version 2.12
© 2004.
Licensed under the GNU Free Documentation License.
http://linear.ups.edu/
In this section we will describe a compact way to indicate the elements of an
infinite set of vectors, making use of linear combinations. This will give us a
convenient way to describe the elements of a set of solutions to a linear
system, or the elements of the null space of a matrix, or many other sets of
vectors.
In Example VFSAL we saw the solution set of a homogeneous system described as all possible linear combinations of two particular vectors. This happens to be a useful way to construct or describe infinite sets of vectors, so we encapsulate this idea in a definition.
Definition SSCV
Span of a Set of Column Vectors
Given a set of vectors  u1
u1 u2
u2 u3
u3 …
… up
up
 S
S
 u2
u2 u3
u3 …
… up
up
 S
S =
= α1u1+α2u2+α3u3+⋯+αpup∣αi∈ℂ
α1u1+α2u2+α3u3+⋯+αpup∣αi∈ℂ 1≤i≤p
1≤i≤p =
= ∑pi=1αiui∣αi∈ℂ
∑pi=1αiui∣αi∈ℂ 1≤i≤p
1≤i≤p
(This definition contains Notation SSV.)
The span is just a set of vectors, though in all but one situation it is an infinite
set. (Just when is it not infinite?) So we start with a finite collection of vectors
 S
S
 S
S
Example ABS
A basic span
Consider the set of 5 vectors,
              1 1 3 1 1 1 3 1            2 1 2 −1 2 1 2 −1            7 3 5 −5 7 3 5 −5            1 1 −1 2 1 1 −1 2            −1 0 9 0 −1 0 9 0               |
and consider the infinite set of vectors
 S
S
 S
S
     1 1 3 1 1 1 3 1      +(1) +(1)     2 1 2 −1 2 1 2 −1      +(−1) +(−1)     7 3 5 −5 7 3 5 −5      +(2) +(2)     1 1 −1 2 1 1 −1 2      +(3) +(3)     −1 0 9 0 −1 0 9 0      = =     −4 2 28 10 −4 2 28 10      |
     1 1 3 1 1 1 3 1      +(−6) +(−6)     2 1 2 −1 2 1 2 −1      +(−3) +(−3)     7 3 5 −5 7 3 5 −5      +(4) +(4)     1 1 −1 2 1 1 −1 2      +(2) +(2)     −1 0 9 0 −1 0 9 0      = =     −26 −6 2 34 −26 −6 2 34      |
     1 1 3 1 1 1 3 1      +(0) +(0)     2 1 2 −1 2 1 2 −1      +(1) +(1)     7 3 5 −5 7 3 5 −5      +(0) +(0)     1 1 −1 2 1 1 −1 2      +(1) +(1)     −1 0 9 0 −1 0 9 0      = =     7 4 17 −4 7 4 17 −4      |
     1 1 3 1 1 1 3 1      +(0) +(0)     2 1 2 −1 2 1 2 −1      +(0) +(0)     7 3 5 −5 7 3 5 −5      +(0) +(0)     1 1 −1 2 1 1 −1 2      +(0) +(0)     −1 0 9 0 −1 0 9 0      = =     0 0 0 0 0 0 0 0      |
The purpose of a set is to collect objects with some common property, and to
exclude objects without that property. So the most fundamental question about a
set is if a given object is an element of the set or not. Let’s learn more about
 S
S
First, is 



 −15 −6 19 5
−15 −6 19 5 




 S
S
 α2
α2 α3
α3 α4
α4 α5
α5
     1 1 3 1 1 1 3 1      +α2 +α2     2 1 2 −1 2 1 2 −1      +α3 +α3     7 3 5 −5 7 3 5 −5      +α4 +α4     1 1 −1 2 1 1 −1 2      +α5 +α5     −1 0 9 0 −1 0 9 0      =u= =u=     −15 −6 19 5 −15 −6 19 5      |
Applying Theorem SLSLC we recognize the search for these scalars as a solution to a linear system of equations with augmented matrix
     1 1 3 1 2 1 2 −1 7 3 5 −5 1 1 −1 2 −1 0 9 0 −15 −6 19 5 1 1 3 1 2 1 2 −1 7 3 5 −5 1 1 −1 2 −1 0 9 0 −15 −6 19 5      |
which row-reduces to
     1 0 0 0 0 1 0 0 −1 4 0 0 0 0 1 0 3 −1 −2 0 10 −9 −7 0 1 0 0 0 0 1 0 0 −1 4 0 0 0 0 1 0 3 −1 −2 0 10 −9 −7 0      |
At this point, we see that the system is consistent
(Theorem RCLS), so we know there is a solution for the five scalars
 α2
α2 α3
α3 α4
α4 α5
α5 S
S
This particular solution allows us to write
     1 1 3 1 1 1 3 1      +(1) +(1)     2 1 2 −1 2 1 2 −1      +(−2) +(−2)     7 3 5 −5 7 3 5 −5      +(−3) +(−3)     1 1 −1 2 1 1 −1 2      +(2) +(2)     −1 0 9 0 −1 0 9 0      =u= =u=     −15 −6 19 5 −15 −6 19 5      |
making it even more obvious that
 S
S
Lets do it again. Is 



 3 1 2 −1
3 1 2 −1 




 S
S
 α2
α2 α3
α3 α4
α4 α5
α5
     1 1 3 1 1 1 3 1      +α2 +α2     2 1 2 −1 2 1 2 −1      +α3 +α3     7 3 5 −5 7 3 5 −5      +α4 +α4     1 1 −1 2 1 1 −1 2      +α5 +α5     −1 0 9 0 −1 0 9 0      =v= =v=     3 1 2 −1 3 1 2 −1      |
Applying Theorem SLSLC we recognize the search for these scalars as a solution to a linear system of equations with augmented matrix
     1 1 3 1 2 1 2 −1 7 3 5 −5 1 1 −1 2 −1 0 9 0 3 1 2 −1 1 1 3 1 2 1 2 −1 7 3 5 −5 1 1 −1 2 −1 0 9 0 3 1 2 −1      |
which row-reduces to
     1 0 0 0 0 1 0 0 −1 4 0 0 0 0 1 0 3 −1 −2 0 0 0 0 1 1 0 0 0 0 1 0 0 −1 4 0 0 0 0 1 0 3 −1 −2 0 0 0 0 1      |
At this point, we see that the system is inconsistent by
Theorem RCLS, so we know there is not a solution for the five scalars
 α2
α2 α3
α3 α4
α4 α5
α5 S
S
Example SCAA
Span of the columns of Archetype A
Begin with the finite set of three vectors of size
 u1 u1 u2 u2 u3 u3 = =     1 2 1 1 2 1      −1 1 1 −1 1 1      2 1 0 2 1 0   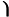 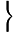 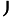 |
and consider the infinite set  S
S
  1 2 1 1 2 1   +(−3) +(−3)  −1 1 1 −1 1 1   +(7) +(7)  2 1 0 2 1 0   = =  22 14 2 22 14 2   |
is in  S
S
 u2
u2 u3
u3 S
S
 α2=−3
α2=−3 α3=7
α3=7 α2
α2 α3
α3
So we know how to quickly construct sample elements of the set
 S
S
 S
S

 1 8 5
1 8 5 

 S
S
 S
S
To answer this question, we will look for scalars
 α2
α2 α3
α3

 1 0 0 0 1 0 1 −1 0 3 2 0
1 0 0 0 1 0 1 −1 0 3 2 0 

 =w
=w =1 =8 =5
=1 =8 =5 By Theorem SLSLC solutions to this vector equation are solutions to the
system of equations Building the augmented matrix for this linear system, and row-reducing,
gives
By Theorem SLSLC solutions to this vector equation are solutions to the
system of equations Building the augmented matrix for this linear system, and row-reducing,
gives This system has infinitely many solutions (there’s a free variable in
tells us that
|
|
so we are convinced that  S
S
shows us that
|
|
Verifying the arithmetic in this second solution will make it obvious that
 S
S

 2 4 3
2 4 3 

 S
S
So we’ll look for scalars  α2
α2 α3
α3

 1 0 0 0 1 0 1 −1 0 0 0 1
1 0 0 0 1 0 1 −1 0 0 0 1 
 =y
=y =2 =4 =3
=2 =4 =3 By Theorem SLSLC solutions to this vector equation are the solutions to the
system of equations Building the augmented matrix for this linear system, and row-reducing,
gives
By Theorem SLSLC solutions to this vector equation are the solutions to the
system of equations Building the augmented matrix for this linear system, and row-reducing,
gives This system is inconsistent (there’s a leading 1 in the
last column, Theorem RCLS), so there are no scalars
 α2
α2 α3
α3 u2
u2 u3
u3 S
S
There are three things to observe in this example. (1) It is easy to construct vectors in
 S
S
 S
S
 S
S
With a computer program in hand to solve systems of linear equations, could you create a program to decide if a vector was, or wasn’t, in the span of a given set of vectors? Is this art or science?
This example was built on vectors from the columns of the
coefficient matrix of Archetype A. Study the determination that
 S
S
Having analyzed Archetype A in Example SCAA, we will of course subject Archetype B to a similar investigation.
Example SCAB
Span of the columns of Archetype B
Begin with the finite set of three vectors of size
 v1 v1 v2 v2 v3 v3 = =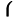     −7 5 1 −7 5 1      −6 5 0 −6 5 0      −12 7 4 −12 7 4      |
and consider the infinite set  R
R
  −7 5 1 −7 5 1   +(4) +(4)  −6 5 0 −6 5 0   +(−3) +(−3)  −12 7 4 −12 7 4   = =  −2 9 −10 −2 9 −10   |
is in  R
R
 v2
v2 v3
v3 R
R
 α2
α2 α3
α3 R
R
Now ask if a given vector is an element of
 R
R

 −33 24 5
−33 24 5 

 R
R
 R
R
To answer this question, we will look for scalars
 α2
α2 α3
α3

 1 0 0 0 1 0 0 0 1 −3 5 2
1 0 0 0 1 0 0 0 1 −3 5 2 

 =z
=z =−33 =24 =5
=−33 =24 =5 By Theorem SLSLC solutions to this vector equation are the solutions to the
system of equations Building the augmented matrix for this linear system, and row-reducing,
gives
By Theorem SLSLC solutions to this vector equation are the solutions to the
system of equations Building the augmented matrix for this linear system, and row-reducing,
gives This system has a unique solution,
telling us that
|
|
so we are convinced that  R
R
Let’s ask about another vector, say is

 −7 8 −3
−7 8 −3 

 R
R
 R
R
We desire scalars  α2
α2 α3
α3

 1 0 0 0 1 0 0 0 1 1 2 −1
1 0 0 0 1 0 0 0 1 1 2 −1 
 =x
=x =−7 =8 =−3
=−7 =8 =−3 By Theorem SLSLC solutions to this vector equation are the solutions to the
system of equations Building the augmented matrix for this linear system, and row-reducing,
gives
By Theorem SLSLC solutions to this vector equation are the solutions to the
system of equations Building the augmented matrix for this linear system, and row-reducing,
gives This system has a unique solution,
telling us that
|
|
so we are convinced that  R
R
We could continue to test other vectors for membership in
 R
R
 R
R
 α2
α2 α3
α3 v2
v2 v3
v3 R
R
 R
R
 R
R =ℂ3
=ℂ3
Compare this example with Example SCAA, and see if you can connect
We saw in Example VFSAL that when a system of equations is homogeneous the
solution set can be expressed in the form described by Theorem VFSLS where the
vector  u2
u2 u3
u3 …
… un−r
un−r
Theorem SSNS
Spanning Sets for Null Spaces
Suppose that  d1
d1 d2
d2 d3
d3 …
… dr
dr
 f1
f1 f2
f2 f3
f3 …
… fn−r
fn−r
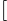 zj zj i= i=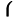      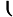 1 0 − 1 0 −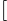 B B k k fj if i∈F, i=fj if i∈F, i≠fj if i∈D, i=dk fj if i∈F, i=fj if i∈F, i≠fj if i∈D, i=dk |
Then the null space of
 A A = =  z1 z1 z2 z2 z3 z3 … … zn−r zn−r   |
Proof Consider the homogeneous system with
 A
A 0
0
 A
A
Now apply Theorem VFSLS to 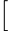 B′
B′ k
k n+1
n+1
 A
A =S =
=S = c+α1u1+α2u2+α3u3+⋯+αn−run−r∣α1
c+α1u1+α2u2+α3u3+⋯+αn−run−r∣α1 α2
α2 α3
α3 …
… αn−r∈ℂ
αn−r∈ℂ =
= α1u1+α2u2+α3u3+⋯+αn−run−r∣α1
α1u1+α2u2+α3u3+⋯+αn−run−r∣α1 α2
α2 α3
α3 …
… αn−r∈ℂ
αn−r∈ℂ =
=
 z1
z1 z2
z2 z3
z3 …
… zn−r
zn−r

Example SSNS
Spanning set of a null space
Find a set of vectors,  S
S =N
=N A
A
     1 2 1 −1 3 5 1 −4 3 7 5 −2 −1 1 1 0 −5 1 5 4 1 2 1 −1 3 5 1 −4 3 7 5 −2 −1 1 1 0 −5 1 5 4      |
The null space of  A
A 0
0
Begin by row-reducing
     1 0 0 0 0 1 0 0 6 −1 0 0 0 0 1 0 4 −2 3 0 1 0 0 0 0 1 0 0 6 −1 0 0 0 0 1 0 4 −2 3 0      |
With  1
1 2
2 4
4
 3
3 5
5
       x1 x2 x3 x4 x5 x1 x2 x3 x4 x5        = =       −6x3−4x5 x3+2x5 x3 −3x5 x5 −6x3−4x5 x3+2x5 x3 −3x5 x5        =x3 =x3       −6 1 1 0 0 −6 1 1 0 0        +x5 +x5       −4 2 0 −3 1 −4 2 0 −3 1        |
Then in the notation of Theorem SSNS,






 −6 1 1 0 0
−6 1 1 0 0 





 z2 =
z2 =





 −4 2 0 −3 1
−4 2 0 −3 1 






and
 A A = =  z1 z1 z2 z2  = =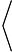 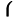              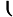        −6 1 1 0 0 −6 1 1 0 0                −4 2 0 −3 1 −4 2 0 −3 1        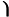       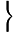        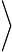 |
Example NSDS
Null space directly as a span
Let’s express the null space of
       2 1 −1 −3 3 1 1 1 2 −1 5 3 −1 −4 5 1 1 0 −4 2 5 6 4 −7 2 1 −1 −3 0 3 2 1 −1 −3 3 1 1 1 2 −1 5 3 −1 −4 5 1 1 0 −4 2 5 6 4 −7 2 1 −1 −3 0 3        |
Theorem SSNS creates vectors for the span by first
row-reducing the matrix in question. The row-reduced version of
       1 0 0 0 0 0 1 0 0 0 2 1 0 0 0 0 0 1 0 0 −1 3 4 0 0 2 −1 −2 0 0 1 0 0 0 0 0 1 0 0 0 2 1 0 0 0 0 0 1 0 0 −1 3 4 0 0 2 −1 −2 0 0        |
We will mechanically follow the prescription of Theorem SSNS. Here we go, in two big steps.
First, the non-pivot columns have indices
 3
3 5
5 6
6

 1 0 0
1 0 0 
 z2 =
z2 =
 0 1 0
0 1 0 
 z3 =
z3 =
 0 0 1
0 0 1 

Each of these vectors arises due to the presence of a column that is not a
pivot column. The remaining entries of each vector are the entries
of the corresponding non-pivot column, negated, and distributed
into the empty slots in order (these slots have indices in the set









 −2 −1 1 0 0 0
−2 −1 1 0 0 0 








 z2 =
z2 =








 1 −3 0 −4 1 0
1 −3 0 −4 1 0 








 z3 =
z3 =








 −2 1 0 2 0 1
−2 1 0 2 0 1 









So, by Theorem SSNS, we have
 A A = =  z1 z1 z2 z2 z3 z3  = =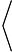 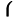                  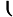           −2 −1 1 0 0 0 −2 −1 1 0 0 0                      1 −3 0 −4 1 0 1 −3 0 −4 1 0                      −2 1 0 2 0 1 −2 1 0 2 0 1           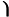                    |
We know that the null space of  A
A 0
0
More advanced computational devices will compute the null space of a matrix. See: Computation NS.MMA Here’s an example that will simultaneously exercise the span construction and Theorem SSNS, while also pointing the way to the next section.
Example SCAD
Span of the columns of Archetype D
Begin with the set of four vectors of size
 w1 w1 w2 w2 w3 w3 w4 w4 = =     2 −3 1 2 −3 1      1 4 1 1 4 1      7 −5 4 7 −5 4      −7 −6 −5 −7 −6 −5      |
and consider the infinite set  T
T
     2 3 0 1 2 3 0 1      |
is a solution to the homogeneous system
 D
D 0
0
|
|
which we can solve for
 |
This equation says that whenever we encounter the vector
 (−2)w1+(−3)w2
(−2)w1+(−3)w2 =5w1+(−4)w2+6w3+
=5w1+(−4)w2+6w3+ 6w1+9w2
6w1+9w2 =11w1+5w2+6w3
=11w1+5w2+6w3
So what began as a linear combination of the vectors
 w2
w2 w3
w3 w4
w4 w2
w2 w3
w3
  w1 w1 w2 w2 w3 w3   |
So the span of our set of vectors,
Check that the vector
     −3 −1 1 0 −3 −1 1 0      |
is a solution to the homogeneous system
 D
D 0
0
|
|
which we can solve for
 |
This equation says that whenever we encounter the vector
  w1 w1 w2 w2   |
So
What was it about the original set of four vectors that allowed us to
declare certain vectors as surplus? And just which vectors were we able to
dismiss? And why did we have to stop once we had two vectors remaining?
The answers to these questions motivate “linear independence,” our next
section and next definition, and so are worth considering carefully now.
It is possible to have your computational device crank out the vector form of the solution set to a linear system of equations. See: Computation VFSS.MMA
     1 2 −1 1 2 −1      3 −4 2 3 −4 2      4 −2 1 4 −2 1      |
Let  S
S

 −1 8 −4
−1 8 −4 


 6 5 −1
6 5 −1 

 S
S =N
=N A
A
 A
A
  1 2 1 3 1 1 1 −3 −1 9 8 5 1 2 1 3 1 1 1 −3 −1 9 8 5   |
C22 For each archetype that is a system of equations, consider the
corresponding homogeneous system of equations. Write elements of the
solution set to these homogeneous systems in vector form, as guaranteed by
Theorem VFSLS. Then write the null space of the coefficient matrix of each
system as the span of a set of vectors, as described in Theorem SSNS.
Archetype A
Archetype B
Archetype C
Archetype D/ Archetype E
Archetype F
Archetype G/ Archetype H
Archetype I
Archetype J
Contributed by Robert Beezer Solution [381]
C23 Archetype K and Archetype L are defined as matrices. Use Theorem SSNS directly
to find a set  S
S
Contributed by Robert Beezer Solution [381]
C40 Suppose that 











 2 −1 3 4
2 −1 3 4 









 3 2 −2 1
3 2 −2 1 







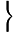



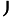
 S
S




 5 8 −12 −5
5 8 −12 −5 




Contributed by Robert Beezer Solution [381]
C41 Suppose that 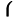







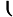




 2 −1 3 4
2 −1 3 4 









 3 2 −2 1
3 2 −2 1 










 S
S




 5 1 3 5
5 1 3 5 




Contributed by Robert Beezer Solution [382]
C42 Suppose 













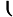






 2 −1 3 4 0
2 −1 3 4 0 













 1 1 2 2 −1
1 1 2 2 −1 













 3 −1 0 3 −2
3 −1 0 3 −2 






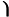






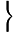






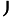






 1 −1 −8 −4 −3
1 −1 −8 −4 −3 






 R
R
Contributed by Robert Beezer Solution [384]
C43 Suppose 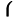













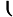






 2 −1 3 4 0
2 −1 3 4 0 













 1 1 2 2 −1
1 1 2 2 −1 













 3 −1 0 3 −2
3 −1 0 3 −2 






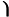






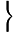












 1 1 5 3 1
1 1 5 3 1 






 R
R
Contributed by Robert Beezer Solution [385]
C44 Suppose that 


 −1 2 1
−1 2 1 



 3 1 2
3 1 2 



 1 5 4
1 5 4 



 −6 5 1
−6 5 1 

 S
S

 −5 3 0
−5 3 0 

Contributed by Robert Beezer Solution [387]
C45 Suppose that 


 −1 2 1
−1 2 1 



 3 1 2
3 1 2 



 1 5 4
1 5 4 



 −6 5 1
−6 5 1 

 S
S

 2 1 3
2 1 3 

Contributed by Robert Beezer Solution [389]
C50 Let
(a) Find a set  A
A =
= S
S
(b) If 



 3 −5 1 2
3 −5 1 2 




 A
A
(c) Write

 2 1 −1 3 2 0 1 1 1 4 3 1
2 1 −1 3 2 0 1 1 1 4 3 1 

Contributed by Robert Beezer Solution [390]
C60 For the matrix  S
S =N
=N A
A
  1 1 −2 1 −2 1 6 0 −6 −8 1 7 1 1 −2 1 −2 1 6 0 −6 −8 1 7   |
Contributed by Robert Beezer Solution [393]
M10 Consider the set of all size
Contributed by Chris Black Solution [394]
M11 Consider the set of all size
Contributed by Chris Black Solution [395]
M12 Let 
 1 3 −2
1 3 −2 


 2 −2 1
2 −2 1 

 u
u v
v w1
w1 =
= u
u v
v
 u
u v
v w2
w2 ≠
≠ u
u v
v
Contributed by Chris Black Solution [396]
M20 In Example SCAD we began with the four columns of the coefficient
matrix of Archetype D, and used these columns in a span construction. Then we
methodically argued that we could remove the last column, then the third
column, and create the same set by just doing a span construction with the first
two columns. We claimed we could not go any further, and had removed as many
vectors as possible. Provide a convincing argument for why a third vector cannot
be removed.
Contributed by Robert Beezer
M21 In the spirit of Example SCAD, begin with the four columns of the coefficient
matrix of Archetype C, and use these columns in a span construction to build the set
Contributed by Robert Beezer Solution [397]
T10 Suppose that  v2∈ℂm
v2∈ℂm
  v1 v1 v2 v2  = =  v1 v1 v2 v2 5v1+3v2 5v1+3v2  |
Contributed by Robert Beezer Solution [398]
T20 Suppose that  S
S
Contributed by Robert Beezer Solution [400]
T21 Suppose that  y∈
y∈ S
S
 S
S
Contributed by Robert Beezer
T22 Suppose that  S
S
 S
S
Contributed by Robert Beezer
C22 Contributed by Robert Beezer Statement [375]
The vector form of the solutions obtained in this manner will involve precisely the
vectors described in Theorem SSNS as providing the null space of the coefficient
matrix of the system as a span. These vectors occur in each archetype in a
description of the null space. Studying Example VFSAL may be of some
help.
C23 Contributed by Robert Beezer Statement [375]
Study Example NSDS to understand the correct approach to this question. The
solution for each is listed in the Archetypes (Appendix A) themselves.
C40 Contributed by Robert Beezer Statement [375]
Rephrasing the question, we want to know if there are scalars
     2 −1 3 4 2 −1 3 4      +α2 +α2     3 2 −2 1 3 2 −2 1      = =     5 8 −12 −5 5 8 −12 −5      |
Theorem SLSLC allows us to rephrase the question again as a quest for solutions to the system of four equations in two unknowns with an augmented matrix given by
     2 −1 3 4 3 2 −2 1 5 8 −12 −5 2 −1 3 4 3 2 −2 1 5 8 −12 −5      |
This matrix row-reduces to
     1 0 0 0 0 1 0 0 −2 3 0 0 1 0 0 0 0 1 0 0 −2 3 0 0      |
From the form of this matrix, we can see that
     2 −1 3 4 2 −1 3 4      +(3) +(3)     3 2 −2 1 3 2 −2 1      = =     5 8 −12 −5 5 8 −12 −5      |
C41 Contributed by Robert Beezer Statement [375]
Rephrasing the question, we want to know if there are scalars
     2 −1 3 4 2 −1 3 4      +α2 +α2     3 2 −2 1 3 2 −2 1      = =     5 1 3 5 5 1 3 5      |
Theorem SLSLC allows us to rephrase the question again as a quest for solutions to the system of four equations in two unknowns with an augmented matrix given by
     2 −1 3 4 3 2 −2 1 5 1 3 5 2 −1 3 4 3 2 −2 1 5 1 3 5      |
This matrix row-reduces to
     1 0 0 0 0 1 0 0 0 0 1 0 1 0 0 0 0 1 0 0 0 0 1 0      |
With a leading 1 in the last column of this matrix (Theorem RCLS) we can
see that the system of equations has no solution, so there are no values for
C42 Contributed by Robert Beezer Statement [376]
Form a linear combination, with unknown scalars, of
       2 −1 3 4 0 2 −1 3 4 0        +a2 +a2       1 1 2 2 −1 1 1 2 2 −1        +a3 +a3       3 −1 0 3 −2 3 −1 0 3 −2        = =       1 −1 −8 −4 −3 1 −1 −8 −4 −3        |
We want to know if there are values for the scalars that make the
vector equation true since that is the definition of membership in
 R
R
       2 −1 3 4 0 1 1 2 2 −1 3 −1 0 3 −2 1 −1 −8 −4 −3 2 −1 3 4 0 1 1 2 2 −1 3 −1 0 3 −2 1 −1 −8 −4 −3        |
Row-reducing the matrix yields,
       1 0 0 0 0 0 1 0 0 0 0 0 1 0 0 −2 −1 2 0 0 1 0 0 0 0 0 1 0 0 0 0 0 1 0 0 −2 −1 2 0 0        |
From this we see that the system of equations is consistent (Theorem RCLS), and
has a unique solution. This solution will provide a linear combination of the vectors
in
C43 Contributed by Robert Beezer Statement [376]
Form a linear combination, with unknown scalars, of
       2 −1 3 4 0 2 −1 3 4 0        +a2 +a2       1 1 2 2 −1 1 1 2 2 −1        +a3 +a3       3 −1 0 3 −2 3 −1 0 3 −2        = =       1 1 5 3 1 1 1 5 3 1        |
We want to know if there are values for the scalars that make the
vector equation true since that is the definition of membership in
 R
R
       2 −1 3 4 0 1 1 2 2 −1 3 −1 0 3 −2 1 1 5 3 1 2 −1 3 4 0 1 1 2 2 −1 3 −1 0 3 −2 1 1 5 3 1        |
Row-reducing the matrix yields,
       1 0 0 0 0 0 1 0 0 0 0 0 1 0 0 0 0 0 1 0 1 0 0 0 0 0 1 0 0 0 0 0 1 0 0 0 0 0 1 0        |
With a leading 1 in the last column, the system is inconsistent (Theorem RCLS), so there
are no scalars  a2
a2 a3
a3
C44 Contributed by Robert Beezer Statement [376]
Form a linear combination, with unknown scalars, of
  −1 2 1 −1 2 1   +a2 +a2  3 1 2 3 1 2   +a3 +a3  1 5 4 1 5 4   +a4 +a4  −6 5 1 −6 5 1   = =  −5 3 0 −5 3 0   |
We want to know if there are values for the scalars that make the
vector equation true since that is the definition of membership in
 S
S
  −1 2 1 3 1 2 1 5 4 −6 5 1 −5 3 0 −1 2 1 3 1 2 1 5 4 −6 5 1 −5 3 0   |
Row-reducing the matrix yields,
  1 0 0 0 1 0 2 1 0 3 −1 0 2 −1 0 1 0 0 0 1 0 2 1 0 3 −1 0 2 −1 0   |
From this we see that the system of equations is consistent (Theorem RCLS), and
has a infinitely many solutions. Any solution will provide a linear combination of the
vectors in
  −1 2 1 −1 2 1   +(−2) +(−2)  3 1 2 3 1 2   +(3) +(3)  1 5 4 1 5 4   +(2) +(2)  −6 5 1 −6 5 1   = =  −5 3 0 −5 3 0   |
C45 Contributed by Robert Beezer Statement [376]
Form a linear combination, with unknown scalars, of
  −1 2 1 −1 2 1   +a2 +a2  3 1 2 3 1 2   +a3 +a3  1 5 4 1 5 4   +a4 +a4  −6 5 1 −6 5 1   = =  2 1 3 2 1 3   |
We want to know if there are values for the scalars that make the
vector equation true since that is the definition of membership in
 S
S
  −1 2 1 3 1 2 1 5 4 −6 5 1 2 1 3 −1 2 1 3 1 2 1 5 4 −6 5 1 2 1 3   |
Row-reducing the matrix yields,
  1 0 0 0 1 0 2 1 0 3 −1 0 0 0 1 1 0 0 0 1 0 2 1 0 3 −1 0 0 0 1   |
With a leading 1 in the last column, the system is inconsistent (Theorem RCLS), so there
are no scalars  a2
a2 a3
a3 a4
a4 S
S
C50 Contributed by Robert Beezer Statement [376]
(a) Theorem SSNS provides formulas for a set
 RREF RREF  1 0 0 0 1 0 −1 1 0 −1 2 0 1 0 0 0 1 0 −1 1 0 −1 2 0   |
 A
A 0
0
 z1
z1 z2
z2




 1 −1 1 0
1 −1 1 0 



 z2 =
z2 =



 1 −2 0 1
1 −2 0 1 




(b) Simply employ the components of the vector
 A
A 0
0
Since each result is zero,  A
A
(c) By Theorem SSNS we know this must be possible (that is the moral of this exercise).
Find scalars
     1 −1 1 0 1 −1 1 0      +α2 +α2     1 −2 0 1 1 −2 0 1      = =     3 −5 1 2 3 −5 1 2      =z =z |
Theorem SLSLC allows us to convert this question into a question about a system of four equations in two variables. The augmented matrix of this system row-reduces to
     1 0 0 0 0 1 0 0 1 2 0 0 1 0 0 0 0 1 0 0 1 2 0 0      |
A solution is
C60 Contributed by Robert Beezer Statement [377]
Theorem SSNS says that if we find the vector form of the solutions to the homogeneous
system  A
A 0
0
  1 0 0 0 1 0 4 2 0 −5 −3 0 1 0 0 0 1 0 4 2 0 −5 −3 0   |
Moving to the vector form of the solutions (Theorem VFSLS), with free variables
     x1 x2 x3 x4 x1 x2 x3 x4      =x3 =x3     −4 −2 1 0 −4 −2 1 0      +x4 +x4     5 3 0 1 5 3 0 1      |
Then with
        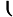      −4 −2 1 0 −4 −2 1 0            5 3 0 1 5 3 0 1               |
Theorem SSNS guarantees that
 A A = = S S = =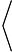 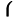        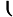      −4 −2 1 0 −4 −2 1 0            5 3 0 1 5 3 0 1      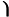    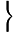     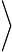 |
M10 Contributed by Chris Black Statement [378]
 v
v =
= αv∣α∈ℝ
αv∣α∈ℝ
 v1 v2
v1 v2 
 0)
0) v2)
v2) u
u v
v =ℝ2
=ℝ2 M11 Contributed by Chris Black Statement [378]
 v
v =
= αv∣α∈ℝ
αv∣α∈ℝ

 v1 v2 v3
v1 v2 v3 

 0
0 0)
0) v2
v2 v3)
v3)
 u1 u1 u1
u1 u1 u1 


 v1 v2 v3
v1 v2 v3 

 u2
u2 u3)
u3) v2
v2 v3)
v3)M12 Contributed by Chris Black Statement [378]
 u
u v
v w1
w1
 u
u v
v

 1 11 −7
1 11 −7 

 u
u v
v

 4 −4 1
4 −4 1 

M21 Contributed by Robert Beezer Statement [379]
If the columns of the coefficient matrix from Archetype C are named
 u2
u2 u3
u3 u4
u4
|
|
by building a homogeneous system of equations and viewing a solution to the system as scalars in a linear combination via Theorem SLSLC. This particular vector equation can be rearranged to read
|
|
This can be interpreted to mean that

 u1
u1 u2
u2 u3
u3 u4
u4

  u1 u1 u2 u2 u3 u3 u4 u4  = =  u1 u1 u2 u2 u3 u3  |
If we try to repeat this process and find a linear combination of
 u2
u2 u3
u3
T10 Contributed by Robert Beezer Statement [379]
This is an equality of sets, so Definition SE applies.
First show that 
 v1
v1 v2
v2
 ⊆
⊆
 v1
v1 v2
v2 5v1+3v2
5v1+3v2
 =Y
=Y
Choose
|
|
which qualifies  v2
v2 5v1+3v2
5v1+3v2
Now show the opposite inclusion, 
 v1
v1 v2
v2 5v1+3v2
5v1+3v2
 ⊆
⊆
 v1
v1 v2
v2
 =X
=X
Choose  a2
a2 a3
a3
|
|
Rearranging, we obtain,
This is an expression for
T20 Contributed by Robert Beezer Statement [380]
No matter what the elements of the set
 v1
v1 v2
v2 v3
v3 …
… vp
vp
But what if we choose